|
|
 |
|
Printable
version of this article in Adobe Acrobat Format
|
Vapor Pressure, An Elegantly
Simple Concept ©
In a later column the workings of a variety of cryopumps will
be discussed. But first, we must understand the mechanisms by
which they pump gases; that is, mechanisms defined as cryocondensation
and cryosorption. These are elegantly simple mechanisms with complex-sounding
names. Cryocondensation ties in closely with the vapor pressure
of a gas, whereas cryosorption relates to the adsorption isotherm
of the gas. We'll return in another article to a discussion of
the latter pumping mechanism. This article deals with the concept
of vapor pressure and how it relates to cryocondensation.
|
|
Cryocondensation involves the surface build-up of thick layers
of either liquids or solids of a gas. For example, water vapor condensation
on the outside of a chilled bottle of Corona beer is just a form
of cryocondensation. Winter ice collecting on an automobile windshield
is another form of water vapor cryocondensation. As long as the
surface is sufficiently cold, and gas continues to impinge on the
surface, the effective pumping of gas on the surface is independent
of the amount of gas previously pumped thereon. Note, however, this
says nothing about the rate that gas might be leaving the surface.
We can define the rate, v ,
at which gas molecules impinge on a surface by the following:
1
|
|
|
|
v =
|
K1 x P
molecules/sec-cm2 (1)
|
|
K1 =
|
3.52 x 10 22 / (MT) ½
|
|
P =
|
the pressure in Torr
|
|
M =
|
the molucular weight of the gas
|
|
T =
|
the temperature of the gas in degrees Kelvin
|
|
R =
|
|
|
It seems reasonable to us all that the rate at which gas impinges
on a surface is directly proportional to the pressure of that gas
above the surface. However, what is less intuitive is that "gas",
in the form of atoms or molecules, is also continuously leaving
all surfaces. And, if there is a lot of a specific material on a
surface, the rate at which that material leaves the surface is dependent
on the vapor pressure of the material. For example, assume that
you have a plate of clean copper on your workbench. The rate at
which copper atoms are leaving or evaporating from the surface of
the plate depends on the vapor pressure of copper at the given temperature.
Now this doesn't necessarily mean that copper atoms are returning
and impinging on the plate. Perhaps the vaporizing copper atoms
are sticking on some other cool surface. By now, you are saying
"so what Kimo?" Stick with me for a moment.
|
|
|
|
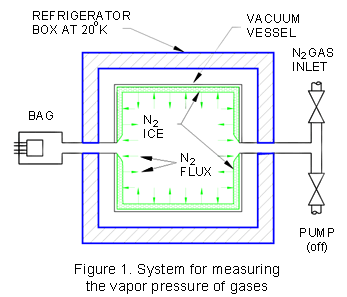
Assume we have an experimental setup as illustrated
in Fig. 1. Assume that the vacuum vessel contained within the refrigerator
box is first evacuated and then cooled to 20 K. We then introduce
nitrogen (N2) gas into the vacuum vessel to the point
where a thick layer of N2"ice" builds up on
the walls of the vacuum vessel, and then shut off the nitrogen valve.
Shortly after shutting off the gas valve, the pressure in the box
equilibrates. That is, the pressure neither increases nor decreases
in the box, but stabilizes at a constant value. If the pressure
is neither increasing nor decreasing, and (1) is valid, this means
that for every molecule of N2 which leaves the ice surface,
one must be impinging on and sticking to the ice. This one-for-one
exchange process suggests that the void within the vessel must be
saturated with N2 gas. It is! And, this is why under
such circumstances we sometimes call that pressure in the vessel
the saturation vapor pressure, or we can simply call it the vapor
pressure of N2 at 20 K. The rate at which the N2
gas is impinging (and departing) the ice can be determined by use
of (1). If we were to slightly increase the temperature of the vessel,
the saturation vapor pressure would equilibrate at a higher value.
If we were to reduce the temperature of the box, the vapor pressure
of N2 gas would correspondingly decrease. Note that if
we attempt to deduce the pressure within the box by using the room
temperature Bayard-Alpert gauge (BAG) outside the box, we must take
into account thermal transpiration effects. But, that's another
subject.
|
| Now, let's
briefly squirt some more N2 gas into the vessel. The pressure
will momentarily increase in the vessel so that the rate at which
the gas molecules impinge on the surface will be greater than the
rate at which they are departing. Eventually, however, the impingement
and departure rates of the gas will again equilibrate, and the net
flux of gas at the surface will again be zero. We say that the introduced
gas has been cryocondensed, or the gas has been pumped by cryocondensation. |
|
Now, let's continually introduce N2 gas into the vessel
so that the pressure in the vessel is always greater than the vapor
pressure of the ice. The gas will continually be pumped on the surface
of the ice. If we know the N2 pressure in the vessel
as a result of continually introducing gas, the rate of impingement
of gas on the 20 K ice can be calculated with (1). On the other
hand, the rate at which the gas is leaving the ice can also be predicted
by (1) by plugging in the value of the vapor pressure of N2
at 20 K.
|
|
Note that if a cold piece of sheet metal was maintained
at 20 K within a room temperature vacuum vessel, the sheet metal
part would just as effectively cryocondense the gas as an equivalent
surface area of the cold wall of our vessel in Fig. 1.
|
|
So, the main thrust of this discussion is that as long as the pressure
of the N2 in the vessel is greater than the vapor pressure
of N2, gas is being pumped on the ice surface. When the
pressure of the N2 gas in the vessel approaches the vapor
pressure of N2, the speed of the ice for N2
approaches zero as the subsequent net accumulation of gas on the
surface will be zero. This is how cryocondensation works. It is
important to note that the vapor pressures of all the gasses, except
He, H2 and Ne, are £
10 -11 Torr at 20K. That is, all those gases are very
effectively cryocondensed at pressures
³ 10 -11 Torr.
It is obvious that knowing the vapor pressure of various gases is
important when trying to predict how well a gas will be pumped (i.e.,
cryocondensed) on a surface of known temperature. Manipulation of
an expression called the Clausius-Clapeyron equation leads to a
simple algebraic equation expressing the vapor pressure, Pv,
of all gases as a function of temperature, T, in degrees
kelvin: 2
|
Log 10 Pv = A
- B x T -1
+ C x Log 10 T (2)
|
Constants
A, B and C vary for the specific gas or material.
Finding the values of these constants was a totally empirical process.
That is, technicians, puttering around in laboratories throughout
the world, made measurements of how different gases behaved as a function
of temperature, compared results, and finally settled on appropriate
values of A, B and C for different gases. Honig
and Hook of RCA were the first to compile a comprehensive listing
of these data for use by the scientific community. 3 If
you know the values of A, B and C, and have the
use of some spreadsheet program (e.g., Lotus, Excel, etc.) you can
plot your own vapor pressure curves, or accurately calculate the vapor
pressure of some gas at a specific temperature. Also, on request many
gas suppliers will provide the values of A, B and C
for some of the esoteric gases which they supply. The values of A,
B and C for some of the common gases are given in Table
1. Have fun making your own vapor pressure curves.
|
Table 1. Clausius-Clapeyron constants
for some of the common gases.
|
|
GAS
|
A
|
B
|
C4
|
|
H2
|
3.881
|
42.730
|
0.50
|
|
D2
|
4.234
|
62.970
|
0.50
|
|
CH4
|
7.123
|
482.871
|
--
|
|
H2O
|
10.285
|
2637.006
|
--
|
|
Ne
|
6.889
|
109.270
|
--
|
|
N2
|
8.362
|
387.428
|
--
|
|
CO
|
8.732
|
445.831
|
--
|
|
O2
|
9.082
|
480.871
|
--
|
|
Ar
|
7.703
|
419.566
|
--
|
|
CO2
|
9.734
|
1349.809
|
--
|
|
Kr
|
7.797
|
581.967
|
--
|
|
Xe
|
7.775
|
801.641
|
--
|
|
The important issue here is that vapor pressure is a very simple concept.
Gases behave according to Equation 1, and the constants A, B and C,
therein, were found by folks around the world puttering in the lab
and trying to fit their experimental results to (1).
|
|
Reference
1 Kimo M. Welch, Capture Pumping Technology, An Introduction,
(Pergamon Press, Oxford 1991), p. 186.
2 Kimo M. Welch, Capture Pumping Technology, An Introduction,
(Pergamon Press, Oxford 1991), p. 255.
3 R.E. Honig and H.O. Hook, "Vapor Pressure Data
for Some Common Gases", RCA Review, 360 (1960).
4 T.J. Lee, "The Condensation of H2 and D2: Astrophysics
and Vacuum Technology", J. Vac. Sci. Technol. 9(1),
257 (1972).
© Copyright Kimo M. Welch February 2000.
|
|

